Autor: Luis Eduardo Munévar Tinoco
This is the human hearing range [20 Hz to 20,000 Hz (20,000 Hz = 20 kHz)] not to be confused with SAMPLING -both the same unit of measurement [Hertz] -.
Simply put the - human hearing range - is the ability to hear sounds in a range between 20 Hz (eg drum (Graves)) to 20 Hz (eg whistle (Acute)) comparative wave is graphically well:
And how is that what Hertz? see:
We can say that the higher the number of Hertz is the sharper sound, and the less most serious Hertz will sound.
Let's see how this made the sound -Wave -:
A cycle time, which has an amplitude forming the wavelength is formed. So if we have a cycle period:
Then FREQUENCY: "Number of times that happens or thing done during a certain period or space."
 y
y
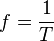
Where T is period.
Then we may have different sounds at different frequencies and amplitudes, then as in the graph of the sound spectrum have wave lengths of 21 meters (16 Hz) and 1.7 cm (20,000 Hz). So here we have the first concept; if we have a sound that is emitted at 20 Hz and we will scan (make binary) for this we need to sample:
Digitization is making a -sampling- at different times of the Wave leg make this data:
Depending on the number of samples taken for each period of the wave will be the sharpness of sound (meaning 'best quality').
This sampling becomes a quantized binary signal:
Now, it is assigned a physical electrical parameters:
In the transition from analog to digital (ADC) there are two sampling processes (number of samples we take the analog signal to more samples, the better representation of the signal) and encoding (the value that we will give all those. samples, the greater the number of bits greater resemblance to the DAC analog signal (step of the digital signal to analog) subsequently appears that our ear is able to hear:
So it is here, where two fundamental concepts are confused:
Here is a sample image sound Digital Analog:
In the case of what we call quality CD; 44.1 kHz: this sampling occurs 44,100 times per second, this 96 kHz sampling occurs 96,000 times per second, this 192 kHz sampling occurs 196,000 times per second.
Amplitude or RESOLUTION
If we increase resolution, sampling frequency, or even both parameters at once by passing an analog signal to digital domain, we can "reconstruct" the original analog signal more accurately, And it really is as follows:
For this reason specifications commonly used in audio formats of high resolution is 24 bits and 96 kHz, or 24 bits and 192 kHz. Both options, on paper, should allow us to recreate the original continuous signal more accurately than the 16-bit, 44.1 kHz CD, or what is the same, discard less information making original sound.
But this is not all. Moreover, resolution up to 24 bits dynamic range increases and improves the signal / noise ratio. A resolution of 16 bits allows us to encode a total of 65,536 possible levels for each of our samples, while a 24-bit reaches 16,777,216 levels, a remarkable difference 260 times.
Read more:
CAN WE APPRECIATE THE DIFFERENCE BETWEEN vinyl record, MP3, CD and high resolution audio?
Fecha: 04 de Julio de 2015
Lima, Perú.
The human ear between 20 hertz (Hz) and 20 000 Hz, some may perceive from 10 Hz to 25000 Hz (or 25 kHz) in acute.This is the human hearing range [20 Hz to 20,000 Hz (20,000 Hz = 20 kHz)] not to be confused with SAMPLING -both the same unit of measurement [Hertz] -.
Simply put the - human hearing range - is the ability to hear sounds in a range between 20 Hz (eg drum (Graves)) to 20 Hz (eg whistle (Acute)) comparative wave is graphically well:
And how is that what Hertz? see:
We can say that the higher the number of Hertz is the sharper sound, and the less most serious Hertz will sound.
Let's see how this made the sound -Wave -:
A cycle time, which has an amplitude forming the wavelength is formed. So if we have a cycle period:
Then FREQUENCY: "Number of times that happens or thing done during a certain period or space."
 y
y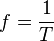
Where T is period.
Then we may have different sounds at different frequencies and amplitudes, then as in the graph of the sound spectrum have wave lengths of 21 meters (16 Hz) and 1.7 cm (20,000 Hz). So here we have the first concept; if we have a sound that is emitted at 20 Hz and we will scan (make binary) for this we need to sample:
Digitization is making a -sampling- at different times of the Wave leg make this data:
Depending on the number of samples taken for each period of the wave will be the sharpness of sound (meaning 'best quality').
This sampling becomes a quantized binary signal:
Now, it is assigned a physical electrical parameters:
In the transition from analog to digital (ADC) there are two sampling processes (number of samples we take the analog signal to more samples, the better representation of the signal) and encoding (the value that we will give all those. samples, the greater the number of bits greater resemblance to the DAC analog signal (step of the digital signal to analog) subsequently appears that our ear is able to hear:
So it is here, where two fundamental concepts are confused:
-Note:- NOT CONFUSE: audible frequency
range of human hearing (20Hz to 20kHz) with sampling (CD quality 44.1 kHz: 96
kHz, 192 kHz) [sampling per second]
|
Here is a sample image sound Digital Analog:
In the case of what we call quality CD; 44.1 kHz: this sampling occurs 44,100 times per second, this 96 kHz sampling occurs 96,000 times per second, this 192 kHz sampling occurs 196,000 times per second.
Clarified that the range of audible
frequencies is not the same as the frequency of sampling per second wave (and
use the same unit of measurement (Hz)), we understand that it is the breadth:
|
Amplitude or RESOLUTION
If we increase resolution, sampling frequency, or even both parameters at once by passing an analog signal to digital domain, we can "reconstruct" the original analog signal more accurately, And it really is as follows:
For this reason specifications commonly used in audio formats of high resolution is 24 bits and 96 kHz, or 24 bits and 192 kHz. Both options, on paper, should allow us to recreate the original continuous signal more accurately than the 16-bit, 44.1 kHz CD, or what is the same, discard less information making original sound.
But this is not all. Moreover, resolution up to 24 bits dynamic range increases and improves the signal / noise ratio. A resolution of 16 bits allows us to encode a total of 65,536 possible levels for each of our samples, while a 24-bit reaches 16,777,216 levels, a remarkable difference 260 times.
Read more:
CAN WE APPRECIATE THE DIFFERENCE BETWEEN vinyl record, MP3, CD and high resolution audio?











No hay comentarios:
Publicar un comentario